Contents
Introduction
The gravitational force is the weakest of the four fundamental forces, yet it is responsible for the interactions of macroscopic objects in the universe such as stars and galaxies due to its infinite range.
In this post, we’re going to explore the characteristics of gravitational fields, including properties such as gravitational force, gravitational field strength and gravitational potential energy.
Let’s begin!
Newton’s law of gravitation
Newton’s law of gravitation tells us about the gravitational force of attraction, \(F\), experienced by a mass \(m\) a displacement \(r\) from a mass \(M\) at the centre of the field:

According to Newton’s law of gravitation, the force of attraction experienced by mass \(m\) is given by:
\(F=\frac{GMm}{r^2}\)
where \(G\) is the gravitational constant. The striking feature of this equation is that it is an inverse square law, with the magnitude of the force decreasing with \(\frac{1}{r^2}\):

We can see from this graph that the force reaches a value of zero when \(r=\infty\), and therefore gravity has an infinite range.
One thing to note is that, while \(r\) points outwards towards \(m\), \(F\) points inwards towards the centre of the field.
Since we define the positive direction to be the same direction as \(r\), this means that \(F\), which points inwards, is negative. However, we often omit the negative sign of the force and just deal with its magnitude. For example, Newton’s law of gravitation and the graph above give the magnitude of \(F\) which of course has positive values.
When dealing with positive values of \(F\), keep in mind therefore that this is the magnitude only.
Radial gravitational fields
Newton’s law of gravitation tells us that the force felt by the mass \(m\) in \(M\)’s gravitational field depends only on \(r\), so the field is radial because it extends into space in the same way in all directions.
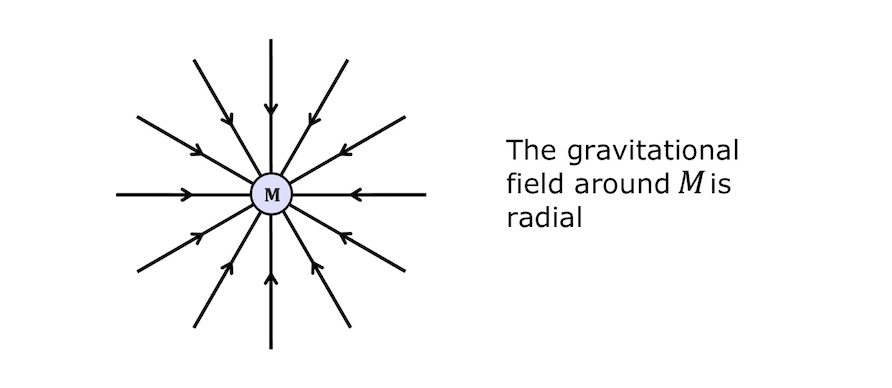
The field lines point inwards because they represent the direction of the field and gravitational fields are attractive.
How to calculate gravitational field strength
An important property of a gravitational field is its field strength, \(g\), at a given point in space. This is defined as the force experienced by unit mass at that point.

It is equal to the gravitational force an object experiences divided by its mass:
\(g=\frac{F}{m}\)
Like gravitational force, gravitational field strength is a vector that points inwards. It therefore takes negative values, so if you see a positive value of gravitational field strength, this is its magnitude.
Substituting Newton’s law of gravitation, \(F=\frac{GMm}{r^2}\), into the definition of \(g\) above, we have the following expression for gravitational field strength:
\(g=\frac{GM}{r^2}\)
This is another inverse square law with gravitational field strength decreasing with \(\frac{1}{r^2}\):

Once again, gravitational field strength reaches zero when \(r=\infty\), meaning that gravity has an infinite range.
How to calculate gravitational potential energy
Another important property is gravitational potential energy. This is the energy stored by a mass \(m\) by virtue of being held at a point in a gravitational field.

There is something intriguing about gravitational potential energy. It is negative! How can an energy be negative?!
To understand this, imagine that an object is so far away from the centre of a gravitational field that it is at \(r=\infty\) and, as such, it is unaffected by the field. It is effectively outside the field. Well, it’s easy to see that for this object the gravitational potential energy is zero because the gravitational field has no effect on it.
Now imagine that we move the object closer to the source of the field to a position \(r<\infty\). The object is now inside the field and affected by it. So its gravitational potential energy is nonzero. But since the gravitational force is always attractive, the object has fallen from \(r=\infty\) to \(r<\infty\), meaning that its gravitational potential energy has decreased. Since its gravitational potential energy started at zero, it must now be negative.
To derive an expression for gravitational potential energy, we can consider the energy change of an object when it moves from \(r=\infty\) to \(r<\infty\). The full derivation involves calculus which you don’t need to know for A Level physics, but it is based on the familiar formula:
\(work\,done=force\times distance\)
combined with Newton’s law of gravitation, \(F=\frac{GMm}{r^2}\).
Since the object “falls” into the field, the work done is negative and the derivation leads to the following negative expression for gravitational potential energy:
\(E=-\frac{GMm}{r}\)
This is an inverse relationship with a negative sign, and a graph of gravitational potential energy against displacement looks like this:

What is gravitational potential?
Like gravitational field strength, gravitational potential, \(V\), is a property of a point in a gravitational field. It is the gravitational potential energy unit mass would have at that position in the field.

It is therefore equal to the gravitational potential energy of an object divided by its mass:
\(V=\frac{E}{m}\)
Since gravitational potential energy is negative, gravitational potential is also negative.
If we substitute in the above equation for gravitational potential energy, we get the following expression for gravitational potential:
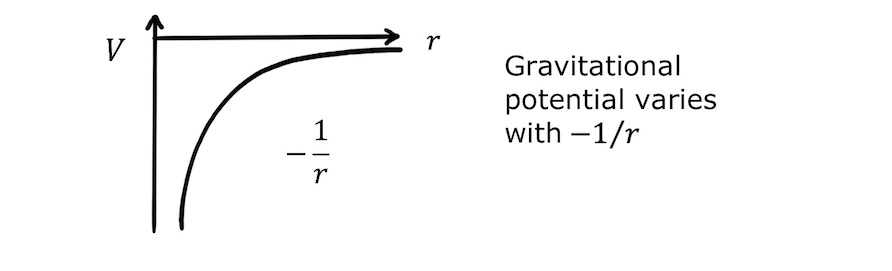
\(V=-\frac{GM}{r}\)
This is another \(-\frac{1}{r}\) relationship which looks like this:
Gravitational equipotential lines
In addition to the field lines we saw above, another useful way of visualising a gravitational field is to use equipotential lines, which represent regions of equal gravitational potential. In a radial field, these are regions of fixed \(r\), which are circles (or, in three dimensions, spheres):
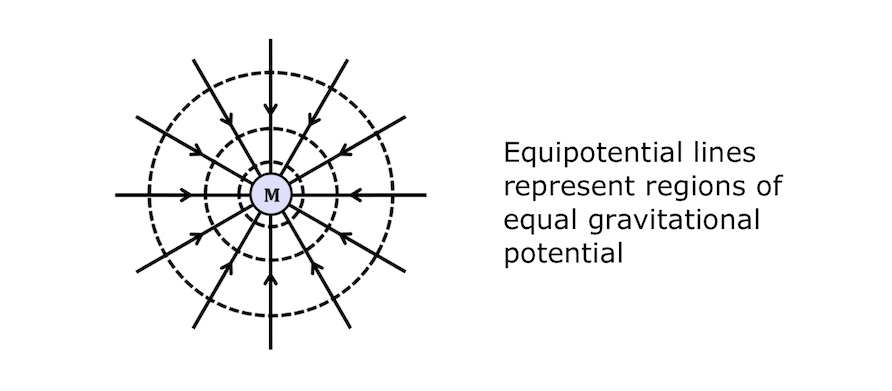
The difference in gravitational potential between adjacent equipotentials is fixed, but as we go further away from the centre of the field the equipotentials are spaced further apart. This is a result of the inverse relationship between \(V\) and \(r\):
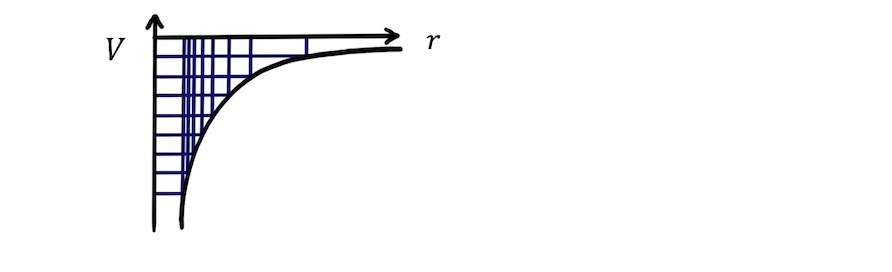
The relationship between gravitational potential and gravitational field strength
In a graph of gravitational potential against displacement, the gradient is equal to the magnitude of the field strength.
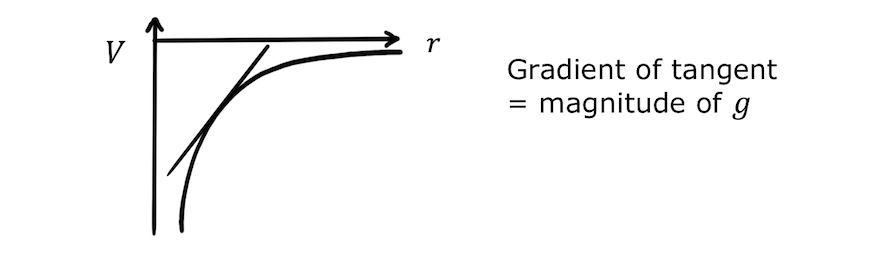
The gradient is positive, but gravitational field strength is negative. So gravitational field strength is equal to minus the gradient:
\(g=-\frac{\Delta V}{\Delta r}\)
This relationship makes intuitive sense. In a region where the field is stronger, more work is required to move unit mass by \(\Delta r\). As a result, \(\frac{\Delta V}{\Delta r}\) is greater. The minus sign is only needed because \(\frac{\Delta V}{\Delta r}\) is positive and \(g\) is negative.
Work done moving a mass in a gravitational field
Let’s look for an expression for the work done moving an object a distance \(\Delta r\) away from the centre of a gravitational field.

We know that the work done is equal to the change in the object’s gravitational potential energy:
\(\Delta E=-\frac{GMm}{\Delta r}\)
Since \(\Delta V=-\frac{GM}{\Delta r}\), we can therefore express work done as:
\(\Delta W=m\Delta V\)
Uniform gravitational fields
So far, we have been considering the radial gravitational field around a mass \(M\). However, at the surface of a sufficiently large object such as a planet, the gravitational field can be approximated as a uniform field.
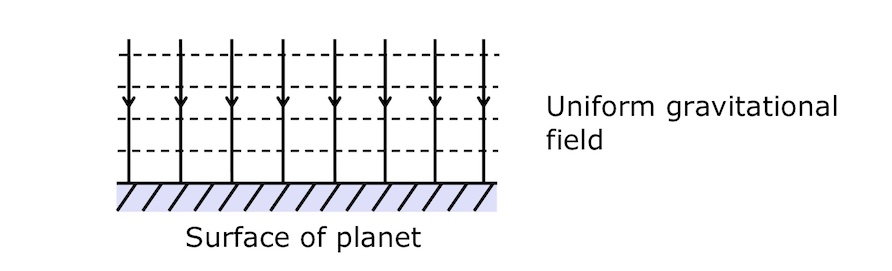
In this approximation, the field lines are parallel (not radial) and the equipotentials are spaced at equal intervals. The equipotentials are equally spaced because \(g=-\frac{\Delta V}{\Delta r}\) is constant.
The force on a given mass is also constant (because \(g\) is constant and \(F=mg\)). And since work done is force multiplied by distance, the work done to raise a mass \(m\) by a height \(h\) is \(\Delta W=F\times d=mg\times h\).
This is our familiar friend: the equation for the work done raising a mass \(m\) by a height \(h\) in a uniform gravitational field:
\(\Delta W=mgh\)
Conclusion
I hope you’ve enjoyed learning about gravitational fields!
We’ve covered Newton’s law of gravitation and some important properties such as gravitational field strength and gravitational potential.
You are now in a great position to tackle the related topic of electric fields and you could even compare gravitational and electric fields with each other.
Happy studying!